I'm reading - well, browsing; or perhaps skipping - Principia Vol 1, and am in the middle of a rather tedious part where he proves various uninteresting results about attractions of spheres and so on in great detail. Section XIV (The motion of very small bodies when agitated by centripetal forces tending to the several parts of any very great body) Proposition XCIV Theorem XLVIII is:
If two similar mediums be separated from each other by a space terminated on both sides by parallel planes, and a body in its passage through that space be attracted or impelled perpendicularly towards either of those mediums, and not agitated or hindered by any other force; and the attraction every where the same at equal distances from either plane, taken towards the same side of the plane: I say, that the sine of incidence upon either plane will be to the sine of emergence from the other plane in a given ratio.
And the proof is (note: you may not want to read the proof, whose details do not concern us, or the scholium that follows; try reading my final para first):

CASE I. Let Aa and Bb be two parallel planes, and let the body light upon the first plane Aa in the direction of the line GH, and in its whole passage through the intermediate space let it be attracted or impelled towards the medium of incidence, and by that action let it be made to describe a curved line HI, and let it emerge in the direction of the line IK. Let there be erected IM perpendicular to Bb the plane of emergence, and meeting the line of incidence GH pro longed in M, and the plane of incidence Aa in R; and let the line of emergence KI be 6 produced and meet HM in L. About the centre L, with the radius LI, let a circle be described cutting both HM in P and Q, and MI produced in N; and, first, if the attraction or impulse be supposed uniform, the curve HI (by what Galileo hath demonstrated) will be a parabola, whose property is that of a rectangle under its given latus rectum, and the line IM equal to iare of HM; and moreover the line HM will be bisected in L. Hence ro MI there be let fall the perpendicular LO, then MOW, OR will be equal; and adding the equal lines ON, Ol, the wholes MN, IR will be equal also. Therefore since IR is given, MN is also given, and the rectangle MI MN is to the rectangle under the latus rectum and IM, that is, to HM in a given ratio. But the rectangle MI MN is equal to the rectangle MP MQ, that is, to the difference of the squares ML2, and PL2 or LI2; and HM2 hath a given ratio to its fourth part ML2; therefore the ratio of ML2-LI2 to ML2 is given, and by conversion the ratio of LI2 to ML2, and its square root, the ratio of LI to ML. But in every triangle, as LMI, the sines of the angles arc proportional to the opposite sides. Therefore the ratio of the sine of the angle of incidence LMR to the sine of the angle of emergence LIR is given. Q.E.D. [Note: in this pic, the planes Ae and Bb are really parallel despite what my rubbish photo might appear to show.]

CASE 2. Let now the body pass successively through several spaces terminated with parallel planes AabB, BbcC, &c., and let it be acted on by a force which is uniform in each of them separately, but different in the different spaces; and by what was just demonstrated, the sine of the angle of incidence on the first plane Aa is to the sine of emergence from the second plane Bb in a given ratio; and this sine of incidence upon the second plane Bb will be to the sine of emergence from the third plane Ce in a given ratio; and this sine to the sine of emergence from the fourth plane Dd in a given ratio; and so on in infinitum, and, by multiplication of equals, the sine of incidence on the first plane is to the sine of emergence from the last plank in a given ratio. Let now the intervals of the planes be diminished, and their number be infinitely increased, so that the action of attraction or impulse, exerted according to any assigned law, may become continual, and the ratio of the sine of incidence on the first plane to the Sine of emergence from the last plane being all along given, will be given then also. Q.E.D.
Aaaanyway, the point is that this is all very dull: who cares other than as an abstract curiosity about motion in such a gap? And it isn't the end of it; he goes on to prove some more. The answer is given by the Scholium at the end:

These attractions bear a great resemblance to the reflections and refractions of light made in a given ratio of the secants, as was discovered by Snell; and consequently in a given ratio of the sines, as was exhibited by Descartes. For it is now certain from the phenomena of Jupiter's satellites, confirmed by the observations of different astronomers, that light is propagated in succession, and requires about seven or eight minutes to travel from the sun to the carth. Moreover, the rays of light that are in our air (as lately was discovered by Grimaldi, by the admission of light in a dark room through a small hole, which I have also tried) in their passage near the angles of bodies, whether transparent or opaque (such as the circular and rectangular edges of gold, silver, and brass coins, or of knives, or broken pieces of stone or glass), are bent or inflected round those bodies as if they were attracted to them; and those rays which in their passage come nearest to the bodies are the most inflected, as if they were most attracted; which thing I myself have also carefully observed. 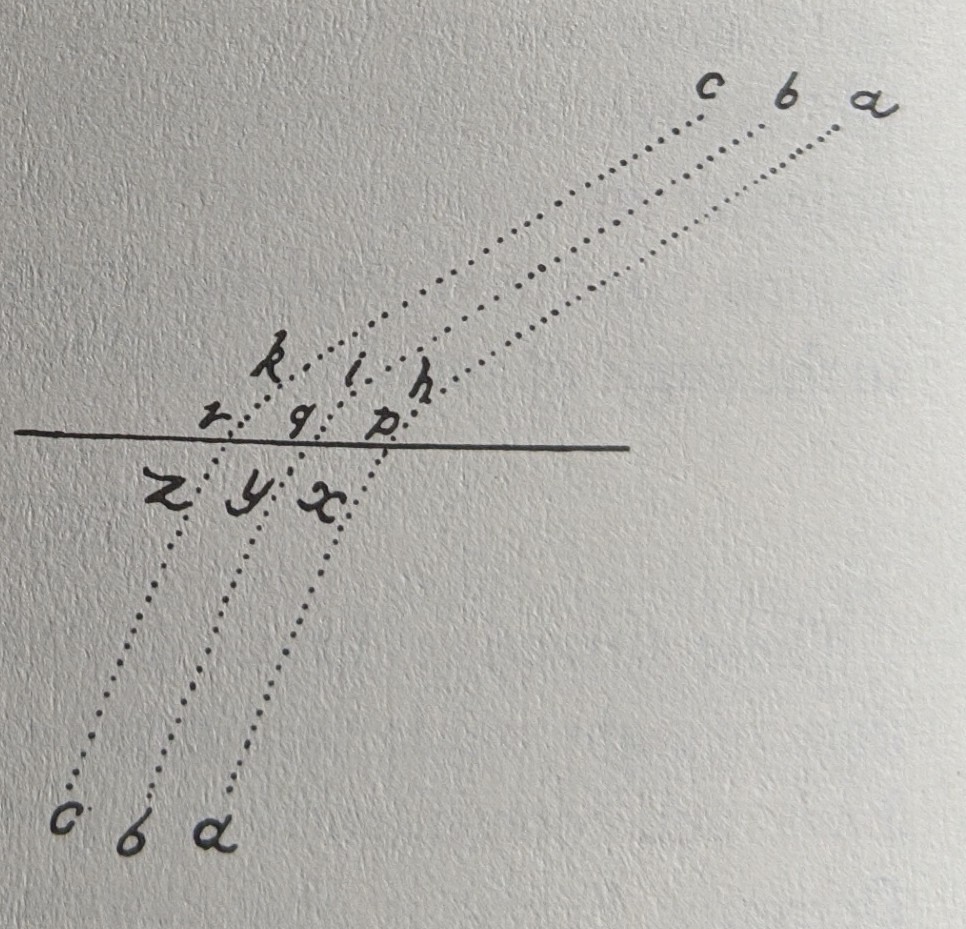 And those which pass at greater distances are less infected; and those at still greater distances are a little inflected the contrary way, and form three fringes of colors. In the figure s represents the edge of a knife, or any kind of wedge AsB; and gowog, fnaf, meme, dsld are rays inflected towards the knife in the arcs owo, non, mom, Ist; which inflection is greater or less according to their distance from the knife. Now since this inflection of the rays is per formed in the air without the knife, it follows that the rays which fall upon the knife are first inflected in the air before they touch the knife. And the case is the same of the rays falling upon glass. The refraction, therefore, is made not in the point of incidence, but grad ually, by a continual inflection of the rays; which is done partly in the air before they touch the glass, partly (if I mistake not) within the glass, after they have entered it; as is represented in the rays cxc, bipi, ahxa, falling upon r, q, p, and inflected between k and z, i and y, h and x. Therefore because of the an alogy there is between the propagation of the rays of light and the motion of bodies, I thought it not amiss to add the following Propositions for optical uses; not at all considering the nature of the rays of light, or inquiring whether they are bodies or not; but only determining the curves of bodies which are extremely like the curves of the rays.
And those which pass at greater distances are less infected; and those at still greater distances are a little inflected the contrary way, and form three fringes of colors. In the figure s represents the edge of a knife, or any kind of wedge AsB; and gowog, fnaf, meme, dsld are rays inflected towards the knife in the arcs owo, non, mom, Ist; which inflection is greater or less according to their distance from the knife. Now since this inflection of the rays is per formed in the air without the knife, it follows that the rays which fall upon the knife are first inflected in the air before they touch the knife. And the case is the same of the rays falling upon glass. The refraction, therefore, is made not in the point of incidence, but grad ually, by a continual inflection of the rays; which is done partly in the air before they touch the glass, partly (if I mistake not) within the glass, after they have entered it; as is represented in the rays cxc, bipi, ahxa, falling upon r, q, p, and inflected between k and z, i and y, h and x. Therefore because of the an alogy there is between the propagation of the rays of light and the motion of bodies, I thought it not amiss to add the following Propositions for optical uses; not at all considering the nature of the rays of light, or inquiring whether they are bodies or not; but only determining the curves of bodies which are extremely like the curves of the rays.
Enough of this Newton clown, what about me?
So, he is trying to understand what will come to be called diffraction (not in the coplanar bit; which is analogous to refraction; I mean in in the bulk of the Scholium). FWIW, this casts some doubt on whether he has indeed observed this stuff himself, or is only relying on others. But more importantly, he's wrong, because diffraction is the wave-like nature of light coming out, and Newton believes in a corpuscular theory. This is minorish; these were early days for a theory of light, people were getting things wrong, and the "true" aka QM view is waaay out of his reach. But nonetheless I think he can know his theory is wrong, by about the one thing he doesn't try to do, which is to try to quantify it - which is common to much of the volume. He knows - or he could know, if he cared to look - that light is not bent to any measureable degree by the gravitational fields of anything as tiny as a piece of glass. But if he admits that, he has no theory at all for diffraction, which would annoy him.
And on the tides
Apparently from nowhere, he pulls out the third power law. This is suspicious (or did I miss something). Did he understand the reason or just somehow guess it? If he understood, I would have expected him to expound on the reasons in some detail. As the book's notes point out, he then tries to deduce the relative density of the moon, but gets it wrong by a factor of two, because he uses the ratio S - L / S + L, but doesn't realise that it is All Very Complicated, especially in channels. But he could have suspected that, and used values from mid-Atlantic islands, perhaps?
And on novae
At the end of the section on comets, he notes that some come very near the sun, and will inevitably eventually fall in. He then speculates that at the other end of their orbits - I think; but he could have meant around other stars; but I think he doesn't realise there's an Ooort cloud, he takes them more generally spread - some fall in, and nourish failing stars, and cause novae. Which is of course wrong, comets are far too small and so on, but it is an interesting idea.
On to Opticks, which is more of a hands-on affair, with N attempting to connect the chemical, physical and optical properties of all the materials he can get his eyes and hands on. The high point may be his obesrvation that since liquid oils yield soot, and are more refractive than water, but less so than solid diamonds, diamond may be "an unctuous Substance much coagulated."
ReplyDeleteThanks. If I'm going to have to cope with the idea that people actually read this stuff I'll have to be more careful :-)
ReplyDeleteHaving read Aristotle's pupil Theophrastus, N may have picked up the idea that silica is a form of water. By the time Lucretius banged out De Rerum Natura, well read folks all agreed quartz crystals from the frigid Alps were just ice frozen so cold that it bloody well stayed that way.
ReplyDeleteThis is known as The Wisdom of the Ancients.